080. Hypothesis Test about the Population Correlation Coefficient

In the case the correlation coefficient is not zero (r ≠ 0) the researcher can conclude on the basis of the sample data that there is a relationship between X and Y. But as always, the interest is with the entire population of all X-values and all Y-values. If the sample data reveal a relationship (r ≠ 0), there may be no such relationship at the population level (ρ = 0).

Therefore it is often desirable to test the hypothesis that the population correlation coefficient is 0. Thus

H0 : ρ = 0;

Hα : ρ ≠ 0.

The hypothesis test is done to determine if it is Significantly different from zero. This test employs the T-statistics

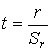, (7.6.5)

(7.6.6)

Has n-2 degrees of freedom, is the standard error of the sampling distribution of R. It recognizes that if several samples of size N were taken, different values for R the researcher would get. If ρ = 0, the r-values would be distributed around ρ, ranging from -1 to +1.

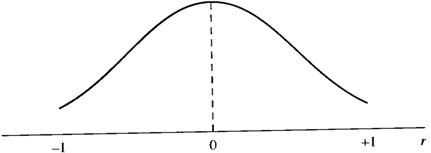
Figure 7.12 – the distribution of Correlation Coefficient R

Then the level of confidence is chosen (for example, 95 percent or α = 0.05) at which the null hypothesis ρ = 0 is tested. This choice allows to find out a critical value of T from the T-table. This critical value of T is compared with the T which was calculated from the Formula (7.6.5) based on the sample data.

In the case the confidence level is 95 percent, and sample size is 15, then it give us 15-2= 13 degrees of freedom and t – values are ±2.160 (Fig.7.13). This means that if ρ = 0, there are only 5 percent chance that the sample would yield a t-value below -2.160 or above +2.160. If t-value from Formula (7.6.5.) is outside the range the researcher can be 95 percent certain that ρ ≠ 0, thus indicating that there is a relationship between X and Y at the population level.

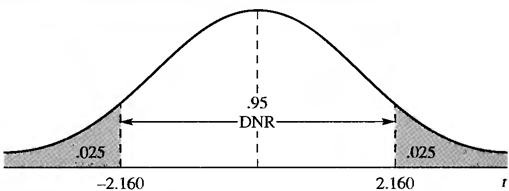
Figure 7.13 – Critical t-Values for Testing the Hypothesis that ρ = 0 (confidence level is 95 percent )

If t-value is between -2.160 and +2.160, then the null hypothesis ρ = 0 cannot be rejected and despite the sample results the researcher would conclude at the 95 percent level of confidence that there is no relationship between X and Y.

< Предыдущая		Следующая >