027. Calculating the Variance and Standard Deviation with Grouped Data
If data are grouped into a frequency table, the variance and standard deviation can be calculated as
 . (3.16)
. (3.16)
And
![]() . (3.17)
. (3.17)
Example 3.9. The flight director for P&P requires information regarding the dispersion of the numbers of passengers. Decisions regarding scheduling and the most efficient size of planes to use depend on the fluctuation in the passenger load. If this variation in number of passengers is large, bigger planes may be needed to avoid overcrowding on those days when the passenger load is extensive. The frequency table for P&P appeared as
Table 3.6 – Frequency Distribution for Passengers
|
Class (passengers) |
Frequency (F) (days) |
Midpoint (M) |
FM |
M2 |
F M2 |
|
50 to 59 |
3 |
54.5 |
163.5 |
2916 |
5,832 |
|
60 to 69 |
7 |
64.5 |
451.5 |
3969 |
19,845 |
|
70 to 79 |
18 |
74.5 |
1341.0 |
5184 |
72,576 |
|
80 to 89 |
12 |
84.5 |
1014.0 |
6561 |
118,098 |
90 to 99 |
8 |
94.5 |
756.0 |
8100 |
56,700 |
|
100 to 109 |
2 |
104.5 |
208.0 |
9801 |
39,204 |
|
∑ |
50 |
3935.0 |
312,255 |
Solution. Given that, the mean was calculated in an earlier example 3.6 as
![]() .
.
Formulas (3.16) and (3.17) give
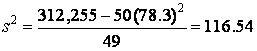 passengers squared,
passengers squared,
![]() passengers.
passengers.
Interpretation. The flight director can now decide if the planes currently in use can accommodate fluctuations in passengers levels as measured by a standard deviation of 10.8. If not, perhaps larger planes will be used to accommodate any overflow that might otherwise occur on those days with heavy traffic.
| < Предыдущая | Следующая > |
|---|